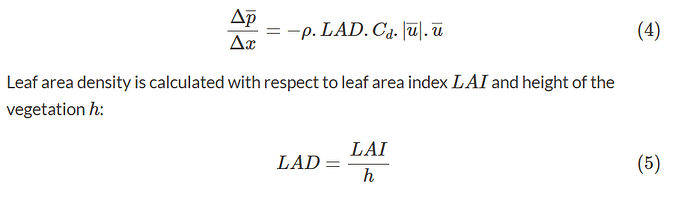Tree model used in PWC

[jjansman]

I have had a few earlier queries in the forum about the used Tree model in PWC. I think I am now starting to get a more consistent story in my head.
I want to verify that and see if some questions I still have can be answered by someone.

In literature (see e.g. here my inventory: Simulating a tree):

• When measuring the drag in e.g. a windtunnel: Cd is depending on wind velocity and on the leaf coverage (LAI) of the tree [Koizuma, 2010, Fig. 5: https://www.researchgate.net/publication/44385014_Evaluation_of_drag_coefficients_of_poplar-tree_crowns_by_a_field_test_method ].
• A leafless tree is around Cd=0.18 (migth be quite velocity indepepdent). And a leafed tree has an average Cd between 0.2 and 1.5 depending on wind velocity [Koizuma, 2010, Fig. 5]

In SIMSCALE PWC doc: Surface Roughness & Porosity | Advanced Modelling PWC | SimScale ad related spreadsheet:

• SIMSCALE uses: fsim = 2LAI/HCdsim
• Cdsim (=0.2) is independant on leaf coverage (LAI) and it looks to be independing on wind velocity.
• Correct?

So questions:

• What Cdsim is to be used (aka how derived from literature Cd’s?)? At what velocity and what leaf coverage?
• What height H is to be used? Is it the tree heigth or the crown height?
• Depending on the above height definition: How would one model a tree with a cylinder: a) of crown height and raised with stem height or b) tree height (not raised). I would think the a) choice would most logical, but this is not shown in the Tree model section of WPC (Figure 10). Sten height quite commenly upto 50% of the tree height.
• Is velocity somewhere included in the fsim?

I hope someone can help me getting a view of how the fsim is defined/derived.

Thanks for your help.

[RicardoParis]

All porous media models that involve polynomial approximations are based on the same formulation, but the formula involves different parameters.

The basic polynomial approximation looks like this:

With C_1 and C_2 being constants, and U velocity.

For Darcy-Forchheimer you’d have something like this:

And for the tree model you’d have what the documentation page that you posted says:

All in all, it’s the same base equation but written with different terms. For the tree model you can see that the Darcy term is zero.

• Cd is assumed, by default, to be fixed at 0.2 for all cases but you can change it with a custom definition.
• Figure 10 in the documentation that you posted shows how to obtain the height
• I normally model a solid trunk (as a solid wall) and a separate volume for the leaves (as a porous media)

Cheers

[jjansman]

Thanks for your answer. I try to use papers/aticles to backup the chosen values, so that is why I come back:

@RicardoParis

• Cd is assumed, by default, to be fixed at 0.2 for all cases but you can change it with a custom definition

Were is this value 0.2 coming from? It looks to be close to the drag coefficient measured for a leafless decidious tree; but is that the origin of the 0.2?

@RicardoParis

• Figure 10 in the documentation that you posted shows how to obtain the height
• I normally model a solid trunk (as a solid wall) and a separate volume for the leaves (as a porous media)

In your case: I assume you use as H, the height of the ‘seperate volume for the leaves’. Correct?

Sorry for this elaboration. But I get the feel, my picture becomes more and more consistent. Thanks.

All the best,