Hi, trying my first transient case I get a steady pressure residual of about 0.28, can´t see where the problem is.
Test case is a box with an inlet and outlet pipe, starts flowing from standstill during 2 seconds.

These are the main parameters for Sim control and numerics. Are there described anywhere? I tweaked them without success to warranty a relative improvement per “inner loop” and a absolute tolerance for the final iteration per timestep (if I undestood well…).
Relaxation factor for field p 0.3
Relaxation factor for equation U 0.7
Number of outer correctors 5 ← not clear what does it mean
Number of correctors 5 ← what is this? iterations at same timestep?
Number of non-orthogonal correctors 1
Pressure reference cell 0
Pressure reference value 0
Correct the flux No
Relaxation factor for field k 0.7
Relaxation factor for field omega 0.7
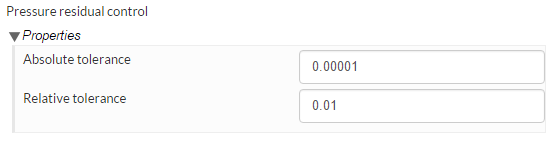
Pressure solver- abs. tolerance 0 rel. tolerance 0.05
Pressure final solver- abs. tolerance 1e-7 rel. tolerance 0
…
Solver Log:
…
ExecutionTime = 3549.78 s ClockTime = 3573 sCourant Number mean: 0.0588417325689 max: 0.698605123499deltaT = 0.00254223447539Time = 1.93135966916PIMPLE: iteration 1DILUPBiCG: Solving for Ux, Initial residual = 0.00067717050359, Final residual = 7.01630659014e-06, No Iterations 1DILUPBiCG: Solving for Uy, Initial residual = 0.000676680997124, Final residual = 3.25648739925e-06, No Iterations 1DILUPBiCG: Solving for Uz, Initial residual = 0.000220470270686, Final residual = 1.04770824031e-06, No Iterations 1DICPCG: Solving for p, Initial residual = 0.26083862015, Final residual = 0.0128328088187, No Iterations 7DICPCG: Solving for p, Initial residual = 0.0201247601123, Final residual = 0.000988426342042, No Iterations 88time step continuity errors : sum local = 2.58509095723e-07, global = -9.84574629e-09, cumulative = -0.000142228323046DICPCG: Solving for p, Initial residual = 0.172548296439, Final residual = 0.00837958242649, No Iterations 7DICPCG: Solving for p, Initial residual = 0.0139416036058, Final residual = 0.000671363205226, No Iterations 89time step continuity errors : sum local = 1.75797767922e-07, global = -1.35255889533e-08, cumulative = -0.000142241848635DICPCG: Solving for p, Initial residual = 0.172482402517, Final residual = 0.00838655106847, No Iterations 7DICPCG: Solving for p, Initial residual = 0.013957980873, Final residual = 0.000669614290432, No Iterations 89time step continuity errors : sum local = 1.75354402065e-07, global = -1.34404299931e-08, cumulative = -0.000142255289065DICPCG: Solving for p, Initial residual = 0.172476812052, Final residual = 0.00839103262979, No Iterations 7DICPCG: Solving for p, Initial residual = 0.0139619675664, Final residual = 0.000676224318838, No Iterations 89time step continuity errors : sum local = 1.77092126734e-07, global = -1.33635544277e-08, cumulative = -0.000142268652619DICPCG: Solving for p, Initial residual = 0.172475121174, Final residual = 0.00838889948961, No Iterations 7DICPCG: Solving for p, Initial residual = 0.0139599759824, Final residual = 0.000671208374209, No Iterations 89time step continuity errors : sum local = 1.75780361693e-07, global = -1.33847841885e-08, cumulative = -0.000142282037403PIMPLE: iteration 2DILUPBiCG: Solving for Ux, Initial residual = 0.000230862225256, Final residual = 9.24944728141e-07, No Iterations 1DILUPBiCG: Solving for Uy, Initial residual = 0.000229791642062, Final residual = 1.12927007786e-06, No Iterations 1DILUPBiCG: Solving for Uz, Initial residual = 7.46074665625e-05, Final residual = 4.70547766495e-06, No Iterations 1DICPCG: Solving for p, Initial residual = 0.168053905339, Final residual = 0.00833821031354, No Iterations 6DICPCG: Solving for p, Initial residual = 0.0127389031591, Final residual = 0.000612620555314, No Iterations 90time step continuity errors : sum local = 1.59164242814e-07, global = -1.45580404022e-08, cumulative = -0.000142296595444DICPCG: Solving for p, Initial residual = 0.112859824716, Final residual = 0.00554343227284, No Iterations 6DICPCG: Solving for p, Initial residual = 0.00884516237995, Final residual = 0.000409448243523, No Iterations 93time step continuity errors : sum local = 1.06492229647e-07, global = -1.86208698096e-08, cumulative = -0.000142315216314DICPCG: Solving for p, Initial residual = 0.112801049958, Final residual = 0.00554351299791, No Iterations 6DICPCG: Solving for p, Initial residual = 0.00884869281756, Final residual = 0.00040605302623, No Iterations 93time step continuity errors : sum local = 1.05624963726e-07, global = -1.84621719217e-08, cumulative = -0.000142333678485DICPCG: Solving for p, Initial residual = 0.112795414624, Final residual = 0.00554726589471, No Iterations 6DICPCG: Solving for p, Initial residual = 0.00885202620444, Final residual = 0.000441219158184, No Iterations 92time step continuity errors : sum local = 1.14778891329e-07, global = -1.75628374635e-08, cumulative = -0.000142351241323DICPCG: Solving for p, Initial residual = 0.112797570301, Final residual = 0.0055496029817, No Iterations 6DICPCG: Solving for p, Initial residual = 0.00885423573902, Final residual = 0.000439548973407, No Iterations 92time step continuity errors : sum local = 1.14345769855e-07, global = -1.75404251375e-08, cumulative = -0.000142368781748PIMPLE: iteration 3DILUPBiCG: Solving for Ux, Initial residual = 9.74337511592e-05, Final residual = 1.01633014915e-06, No Iterations 1DILUPBiCG: Solving for Uy, Initial residual = 9.66201322149e-05, Final residual = 8.98606260137e-07, No Iterations 1DILUPBiCG: Solving for Uz, Initial residual = 3.03794753981e-05, Final residual = 2.34876768988e-07, No Iterations 1DICPCG: Solving for p, Initial residual = 0.109610294541, Final residual = 0.00547950776607, No Iterations 5DICPCG: Solving for p, Initial residual = 0.00780181232291, Final residual = 0.000385452749946, No Iterations 96time step continuity errors : sum local = 9.95931685658e-08, global = -2.1167702001e-08, cumulative = -0.00014238994945DICPCG: Solving for p, Initial residual = 0.0745798976194, Final residual = 0.00369385136774, No Iterations 5DICPCG: Solving for p, Initial residual = 0.00542485409894, Final residual = 0.000248124902362, No Iterations 177time step continuity errors : sum local = 6.41672031837e-08, global = -1.09069525043e-11, cumulative = -0.000142389960357DICPCG: Solving for p, Initial residual = 0.0737924707288, Final residual = 0.00365076436865, No Iterations 5DICPCG: Solving for p, Initial residual = 0.00537055310656, Final residual = 0.000261809472139, No Iterations 96time step continuity errors : sum local = 6.83764321441e-08, global = -1.44243698484e-08, cumulative = -0.000142404384727DICPCG: Solving for p, Initial residual = 0.0743198540053, Final residual = 0.00368518421105, No Iterations 5DICPCG: Solving for p, Initial residual = 0.00541356467056, Final residual = 0.000268882190937, No Iterations 176time step continuity errors : sum local = 6.97400703743e-08, global = 1.9327140544e-10, cumulative = -0.000142404191455DICPCG: Solving for p, Initial residual = 0.073797419797, Final residual = 0.00365329043978, No Iterations 5DICPCG: Solving for p, Initial residual = 0.00537280661118, Final residual = 0.000262636869524, No Iterations 96time step continuity errors : sum local = 6.8585743172e-08, global = -1.44772393998e-08, cumulative = -0.000142418668695PIMPLE: iteration 4DILUPBiCG: Solving for Ux, Initial residual = 5.11468792423e-05, Final residual = 6.22052903696e-07, No Iterations 1DILUPBiCG: Solving for Uy, Initial residual = 5.06606415917e-05, Final residual = 5.51056299633e-07, No Iterations 1DILUPBiCG: Solving for Uz, Initial residual = 1.49111345734e-05, Final residual = 1.13289978527e-07, No Iterations 1DICPCG: Solving for p, Initial residual = 0.0720743455083, Final residual = 0.00307886521672, No Iterations 5DICPCG: Solving for p, Initial residual = 0.0047398410707, Final residual = 0.000235625125893, No Iterations 176time step continuity errors : sum local = 6.07818929628e-08, global = 5.9772860428e-12, cumulative = -0.000142418662718DICPCG: Solving for p, Initial residual = 0.0490839043748, Final residual = 0.0020781884575, No Iterations 5DICPCG: Solving for p, Initial residual = 0.00326977259385, Final residual = 0.000161434113085, No Iterations 177time step continuity errors : sum local = 4.20399036266e-08, global = -4.69035020053e-10, cumulative = -0.000142419131753DICPCG: Solving for p, Initial residual = 0.049066497006, Final residual = 0.0020796949329, No Iterations 5DICPCG: Solving for p, Initial residual = 0.00327303467306, Final residual = 0.000163417741286, No Iterations 179time step continuity errors : sum local = 4.25487254363e-08, global = -9.20300141137e-10, cumulative = -0.000142420052053DICPCG: Solving for p, Initial residual = 0.0490689722987, Final residual = 0.0020809380721, No Iterations 5DICPCG: Solving for p, Initial residual = 0.00327376295612, Final residual = 0.000162704803703, No Iterations 203time step continuity errors : sum local = 4.23604701259e-08, global = -3.10427176063e-10, cumulative = -0.00014242036248DICPCG: Solving for p, Initial residual = 0.0490675631085, Final residual = 0.00208068299735, No Iterations 5DICPCG: Solving for p, Initial residual = 0.00327352655394, Final residual = 0.000156857625855, No Iterations 177time step continuity errors : sum local = 4.0840931101e-08, global = -4.3685499717e-10, cumulative = -0.000142420799335PIMPLE: iteration 5DILUPBiCG: Solving for Ux, Initial residual = 4.36351074985e-05, Final residual = 1.05810947501e-06, No Iterations 1DILUPBiCG: Solving for Uy, Initial residual = 4.31542944718e-05, Final residual = 9.39886200428e-07, No Iterations 1DILUPBiCG: Solving for Uz, Initial residual = 1.27041371012e-05, Final residual = 1.7212989725e-07, No Iterations 1DICPCG: Solving for p, Initial residual = 0.19925535684, Final residual = 0.00921167495846, No Iterations 7DICPCG: Solving for p, Initial residual = 0.0211370181632, Final residual = 0.00102437583511, No Iterations 88time step continuity errors : sum local = 2.54313768143e-07, global = 2.64775747689e-09, cumulative = -0.000142418151577DICPCG: Solving for p, Initial residual = 0.00809156400505, Final residual = 0.00039534549904, No Iterations 23DICPCG: Solving for p, Initial residual = 0.00107205603933, Final residual = 5.2255932566e-05, No Iterations 191time step continuity errors : sum local = 1.28666768962e-08, global = 1.79296652813e-10, cumulative = -0.000142417972281DICPCG: Solving for p, Initial residual = 0.000817880278234, Final residual = 3.96852712979e-05, No Iterations 30DICPCG: Solving for p, Initial residual = 9.52355546567e-05, Final residual = 4.45140291837e-06, No Iterations 195time step continuity errors : sum local = 1.07174128772e-09, global = 2.04157778904e-12, cumulative = -0.000142417970239DICPCG: Solving for p, Initial residual = 9.51422305492e-05, Final residual = 4.55776409886e-06, No Iterations 7DICPCG: Solving for p, Initial residual = 7.39377842642e-06, Final residual = 3.58555413841e-07, No Iterations 206time step continuity errors : sum local = 8.65552468387e-11, global = 1.06930941732e-12, cumulative = -0.00014241796917DICPCG: Solving for p, Initial residual = 1.01329765754e-05, Final residual = 4.76359018717e-07, No Iterations 6DICPCG: Solving for p, Initial residual = 8.42889196851e-07, Final residual = 9.70790719976e-08, No Iterations 25time step continuity errors : sum local = 2.34383458815e-11, global = 1.30917727482e-12, cumulative = -0.000142417967861DILUPBiCG: Solving for omega, Initial residual = 0.000104256674898, Final residual = 8.77058854145e-07, No Iterations 1DILUPBiCG: Solving for k, Initial residual = 0.00118348516952, Final residual = 3.65467230944e-06, No Iterations 2ExecutionTime = 3554.29 s ClockTime = 3578 sregIOobject::readIfModified() : Re-reading object controlDict from file "system/controlDict"Courant Number mean: 0.0588593412964 max: 0.698644190216deltaT = 0.00254223447539Time = 1.93390190364PIMPLE: iteration 1DILUPBiCG: Solving for Ux, Initial residual = 0.000676439757255, Final residual = 6.98632492088e-06, No Iterations 1DILUPBiCG: Solving for Uy, Initial residual = 0.000676091899832, Final residual = 3.22835339646e-06, No Iterations 1DILUPBiCG: Solving for Uz, Initial residual = 0.000220311649741, Final residual = 1.04791680268e-06, No Iterations 1DICPCG: Solving for p, Initial residual = 0.260841984527, Final residual = 0.0128160384365, No Iterations 7DICPCG: Solving for p, Initial residual = 0.0201097630522, Final residual = 0.000993748678274, No Iterations 88time step continuity errors : sum local = 2.60020830834e-07, global = -9.81709555841e-09, cumulative = -0.000142427784956DICPCG: Solving for p, Initial residual = 0.172548480378, Final residual = 0.00836800226039, No Iterations 7DICPCG: Solving for p, Initial residual = 0.0139308396223, Final residual = 0.000672381120172, No Iterations 89time step continuity errors : sum local = 1.76144842506e-07, global = -1.35198357765e-08, cumulative = -0.000142441304792DICPCG: Solving for p, Initial residual = 0.172483279818, Final residual = 0.00837454814298, No Iterations 7DICPCG: Solving for p, Initial residual = 0.0139468168216, Final residual = 0.000672319201463, No Iterations 89time step continuity errors : sum local = 1.76143223259e-07, global = -1.34247636262e-08, cumulative = -0.000142454729556DICPCG: Solving for p, Initial residual = 0.172477246545, Final residual = 0.00837925716192, No Iterations 7DICPCG: Solving for p, Initial residual = 0.013950990446, Final residual = 0.000676260951986, No Iterations 89time step continuity errors : sum local = 1.77182708986e-07, global = -1.33631245007e-08, cumulative = -0.00014246809268DICPCG: Solving for p, Initial residual = 0.17247576963, Final residual = 0.00837813273622, No Iterations 7DICPCG: Solving for p, Initial residual = 0.013949877448, Final residual = 0.000671973441343, No Iterations 89time step continuity errors : sum local = 1.7606135292e-07, global = -1.33757366164e-08, cumulative = -0.000142481468417PIMPLE: iteration 2DILUPBiCG: Solving for Ux, Initial residual = 0.000230631575234, Final residual = 9.17008043666e-07, No Iterations 1
 Keep the flow running!
Keep the flow running!