Documentation
The drag coefficient is a fundamental concept in fluid dynamics that plays a crucial role in quantifying the resistance an object experiences when moving through a fluid medium. Whether it’s an airplane soaring through the sky, a truck cruising down the road, or a dolphin swimming through the ocean, the drag coefficient governs the force interaction between the object and the fluid in the streamwise direction, influencing its motion and efficiency.
In this blog post, we will delve into the history, derivation, usage, and significance of drag coefficient in Computational Fluid Dynamics (CFD) simulations.
The drag coefficient \((C_D)\) is a dimensionless value that helps in quantifying the drag on an object moving through a fluid, representing the object’s aerodynamic or hydrodynamic properties. It is used in modeling drag dependencies on aspects like the object’s shape, inclination, and surrounding flow conditions. Simply put, it measures the effectiveness of a streamline aerodynamic body shape in reducing the resistance of air (or surrounding fluid) to the body’s forward movement through the medium.
For example, a typical passenger car would have a drag coefficient of about \(C_D=0.3\), given its streamlined shape, while the drag coefficient of a bus can go up to \(C_D=0.8\) with a rather box shape. In other words, the air resistance experienced by the car is less than that experienced by the bus.
Drag is, by definition, a force acting through the center of pressure of an object parallel to the direction of the incoming flow. It is generated as a result of the velocity difference between the solid body and the fluid. That means drag is only generated upon relative movement between a body and a fluid. Quantifying this drag force entails the use of the drag coefficient.
The notion of drag has been recognized for centuries, with early observations made by Leonardo da Vinci, who described the resistance experienced by objects moving through air or water. However, the formal concept of drag coefficient was mainly developed in the 19th century, as scientists sought to quantify the resistance of practical objects to aid in efficiency improvements for transport or construction.
In 1883, the German engineer Ludwig Prandtl introduced the concept of drag coefficient \((C_D)\) as a dimensionless quantity that characterizes the force acting on an object parallel to the direction of flow. His work laid the foundation for understanding and quantifying the complex relationship between an object’s shape, the fluid it moves through, and the resulting forces.
The drag coefficient is derived from the drag equation, expressed as:
$$ D = \frac{1}{2} \rho U^2 C_D A $$
Where:
As one can see from the above equation, the drag coefficient is a dimensionless parameter that scales the magnitude of the drag force for a given flow (density and velocity) and object (projected area). The drag coefficient depends on various factors, such as the object’s shape, surface roughness, and the properties of the fluid.
Drag coefficient \(C_D\) is then expressed as:
$$ C_D = \frac{2D}{\rho U^2 S} $$
In general, the shape of an object has a significant impact on its drag coefficient. Different object shapes interact with the surrounding fluid in unique ways, resulting in varying levels of drag. Here are some common shapes and their effects on the drag coefficient:
Streamlined or aerodynamic shapes, such as teardrops or airfoils, are designed to minimize drag. These shapes smoothly guide the fluid around the object, reducing turbulence and pressure variations. As a result, streamlined objects tend to have lower drag coefficients, making them more efficient in terms of reducing resistance and improving speed and fuel economy. This design approach is widely used in aircraft, cars, high-speed rail (HSR), and other high-speed vehicles.
Blunt objects (or bluff bodies), like cubes or spheres, create a lot of drag due to their large cross-sectional area perpendicular to the flow direction and the tendency for the flow to “separate” off of the rear of the object. The abrupt change in shape leads to significant pressure differences and boundary separation, leading to turbulence and increased drag forces. However, blunt shapes may be intentionally used for specific or practical purposes, such as reducing supersonic shockwaves or for stability in certain situations.
The surface roughness of an object can influence its drag coefficient. Rough surfaces create more turbulence and lead to increased skin friction drag. In practical applications, surface treatments like laminar flow control or adding a streamlined fairing can be employed to reduce surface roughness and, in turn, the drag coefficient. However, somewhat unintuitively, upstream surface roughness can lead to a more energetic, turbulent boundary layer near the surface which can delay flow separation and decrease drag for certain shapes (like a golf ball).
Objects with protruding features or appendages experience higher drag due to the additional turbulence they create. Consider an airplane with landing gear extended; the exposed landing gear contributes to higher drag. In some cases, such as Formula 1 cars, the addition of wings and spoilers can be used to intentionally increase drag for enhanced downforce and better traction. Over the years, these winglets have become more integrated into the overall body to eliminate draggy support structures and mounts.
Understanding how object shape affects drag allows engineers and designers to optimize the performance of various vehicles and objects, taking into account the trade-offs between speed, stability, practical matters, and energy consumption.
In the design of aircraft, the drag coefficient is crucial. Engineers strive to minimize drag to enhance fuel efficiency and optimize performance. The shape of the wings, fuselage, and other components are carefully designed to achieve low drag coefficients, allowing planes to reach higher speeds with less fuel consumption. Furthermore, the balance and relationship between the lift and drag coefficients are very important, given the aircraft’s specific purpose.
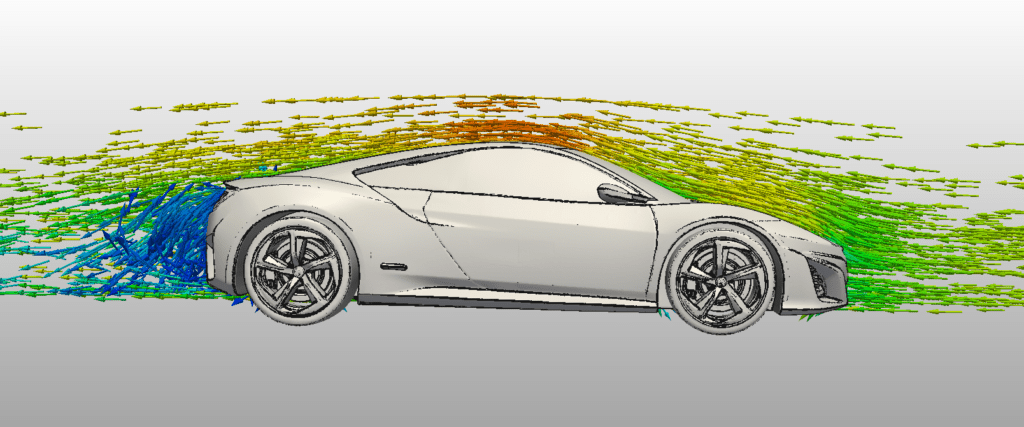
Drag coefficient plays a significant role in designing cars that are more fuel-efficient. Studies and simulations have shown that at highway speeds (typically above 50 mph or 80 km/h), aerodynamic drag becomes a dominant factor in an electric vehicle’s overall energy consumption. In some cases, it can account for around 30% to 50% of the total energy required to propel the vehicle at those speeds.
It’s important to note that aerodynamic drag has a non-linear relationship with speed, meaning that its impact on efficiency increases significantly as the vehicle goes faster. At lower speeds, other factors like rolling resistance and drivetrain losses may have a more prominent influence on efficiency. As a result, the percentage contribution of aerodynamic drag to overall efficiency tends to increase as the vehicle accelerates to higher speeds.
To mitigate the impact of aerodynamic drag on a vehicle’s efficiency, automotive engineers and designers use various techniques such as streamlining the vehicle’s shape, optimizing the underbody, reducing the frontal area, and employing aerodynamic features like spoilers and active grille shutters. All these changes can be represented by a corresponding drag coefficient. By making these improvements, manufacturers can enhance the vehicle’s energy efficiency and extend its driving range, making it more appealing to consumers.
Drag coefficient is vital in sports where athletes are dealing with air resistance, such as cycling, bobsledding, skiing, and motorsports. Designing equipment and clothing to minimize drag helps athletes achieve better performance and gain a competitive edge.
Significant R&D investments are made to continually improve the aerodynamic performance of many pieces of familiar equipment, such as cycling helmets, golf balls, archery equipment, swimming gear, ski suits, and rowing equipment.
In Computational Fluid Dynamics (CFD) simulations, the drag coefficient for a given object or product can be directly predicted as an outcome of the simulation. CFD software utilizes numerical methods to solve the governing viscous fluid flow equations, allowing engineers to visualize and predict the behavior of complex fluid interactions and predict pressure and viscous forces.
By integrating the distribution of the pressures and viscous (shear) forces along the surface, all the overall forces and moments can be calculated. The drag coefficient would be one such force (in addition to lift and lateral forces) that will be a result. CFD enables optimization of designs for improved performance and efficiency without the need for time-intensive and costly physical prototypes and testing.
The drag coefficient is a crucial concept in fluid dynamics that quantifies the resistance an object experiences when moving through a fluid. In the realm of Computational Fluid Dynamics (CFD) simulations, drag coefficient estimation plays a vital role in optimizing designs, reducing costs, and improving performance. Understanding the drag coefficient allows engineers to create more efficient and streamlined designs that harness the power of fluid dynamics to their full advantage.
SimScale’s CFD tool offers engineers the ability to analyze drag phenomena and compute the corresponding drag coefficient of their designs in order to optimize and innovate quickly and efficiently. With SimScale’s cloud-native platform, engineers and designers can conduct their simulations directly in their web browser anytime, anywhere, without the need for any hardware or installation. Simply sign up to the platform, import your CAD design, and simulate away!
Furthermore, for analyses with varying parameters, you can simultaneously run multiple simulations in parallel, which reduces your design cycle time significantly. Not only does SimScale offer accessibility and time efficiency, but it also allows teams to collaborate on projects simultaneously by simply sharing the project with the team members. Make use of SimScale’s CFD capabilities by signing up below.
Last updated: September 1st, 2023
We appreciate and value your feedback.
What's Next
What is Lift Coefficient?Sign up for SimScale
and start simulating now