Documentation
Net positive suction head (NPSH) is a term used in hydraulic system design and, specifically, pump selection. NPSH measures the margin a working liquid has over its vapor phase transition. It provides a definition of the operating conditions that would lead to vapor transition (flashing) and enables us to avoid them, accordingly. In this article, we will explain how engineers have conveniently defined the NPSH Available (NPSHA) at the pump inlet and NPSH Required (NPSHR) for a given pump and how the two relate to one another.
NPSH is used in the design of pumps and hydraulic systems as a key driving parameter governing whether or not cavitation will occur within the pump. Cavitation occurs when the local inlet pressure falls too low, causing pockets of low-pressure vapor to form in the fluid stream. These vapor bubbles can collapse violently against pump components, resulting in micro jet formations that can cause significant damage to both the pump itself and possibly other downstream equipment.

In the most general sense, NPSH is the difference between the pump inlet suction (total) pressure and vapor pressure:
Where
Pt,in = Stagnation (total) pressure at the pump inlet, with the pump running
Pvap = Vapor pressure of the working fluid at the local (inlet) temperature
Pt,in should be expressed in absolute units to be consistent with the vapor pressure, so atmospheric pressure (14.7 psia or 101.325 kPa) should be added to the gauge pressure value.
Finally, the above expression calculates NPSH in pressure units. To convert to head (feet or meters), the below conversions should be applied:
There are a few nuances to gathering accurate input pressures to the above equation. First, the suction total pressure must be determined at the pump inlet nozzle when the pump is running. If the pressure gauge is located above or below the inlet, the hydrostatic pressure based on the height difference must be added or subtracted (respectively). While it is often much smaller than the static pressure head, the velocity (dynamic) head in the pipe at the gauge connection should be added to obtain total (stagnation) pressure.
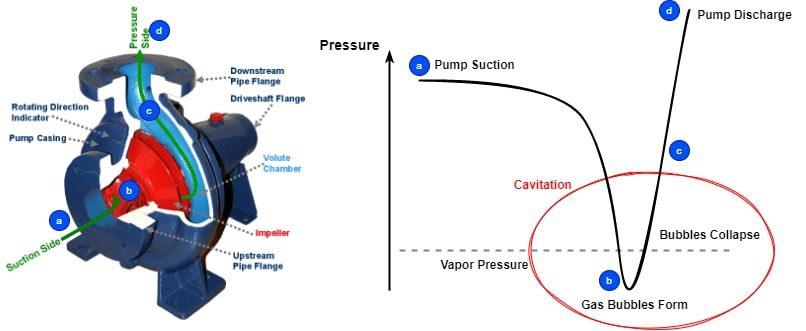
The standard practice in mechanical engineering is to separately break NPSH out into two distinct definitions, NPSHA (available) and NPSHR (required).
NPSHA is the NPSH available to the pump from the system, and it depends solely on the operating environment and the conditions of the fluid being pumped. Cavitation is more likely at higher temperatures since vapor pressure increases with temperature. Therefore, NPSHA tends to decrease as temperature increases. NPSHA can be thought of as a system parameter and not a function of the pump in question.
NPSHR, on the other hand, is determined by the pump. It is the NPSH required by the pump to operate effectively and avoid cavitation, and it is a function of many pump characteristics. Design aspects such as the number and shape of the impeller vanes, impeller eye diameter, speed, shaft and impeller hub diameter, and surface roughness can all affect NPSHR.
To ensure NPSH requirements are met and cavitation is avoided, NPSHR must be greater than NPSHA by a sufficient margin. This margin will ensure that the pump will operate safely over its service life and across a range of fluid conditions. A typical margin is about 10-30% (margin ratio of 1.1-1.3), but specific NPSH requirements will depend on the pump and fluid systems in question.
It is often asked if Net Positive Suction Head (either available or required) can be negative, which sounds counterintuitive given its name. While it is possible for NPSHA to be negative, for all practical purposes, it is not something that we would encounter. A negative NPSHA would mean that the liquid being pumped is boiling as it reaches the pump, which would be very undesirable and you would likely “vapor lock” the pump. Also, since NPSHR is always positive, NPSHA must also be positive (greater) to avoid cavitation in the pump, so it is a moot point.
As previously noted, sufficient Net Positive Suction Head margin between the available and required NPSH must be maintained in order to avoid damaging cavitation. This makes the selection and installation of the pump a vital step in designing a hydraulic system. Once a given pump is selected, there could be some unforeseen changes that lead to insufficient margin in the system. This is common, as projects rarely go to plan, and engineers must be ready to adapt.
There are four steps that can be taken to prevent the onset of pump cavitation:
If all of these mitigation steps have been exhausted, the only remaining choice would be to opt for a different pump with a lower NPSHR.
CFD simulation makes it possible to predict the flow conditions inside a full hydraulic system, providing valuable information for NPSH calculations. These simulations can be carried out at both the system and component levels, depending on the problem one is looking to solve.
From the viewpoint of the engineer designing a fluid system, simulation results are used to determine the NPSHA available at the pump location. NPSHA modeling would consist of a steady-state, single-phase, incompressible fluid simulation of the full hydraulic system. Thermal transport also may be considered in this analysis. CFD can be used to accurately predict the local inlet total pressure and vapor pressure at the pump inlet, which can then be used in the NPSH equation.
For engineers designing the next generation of pump technology, CFD provides valuable visualization and information about the pump’s hydraulic design. Parametric studies can be used to optimize the efficiency and operating envelope of a pump. Advanced pump modeling techniques, such as the SimScale turbomachinery solver, capture the level of cavitation in the pump and can be used to accurately predict the NPSHR. The pump designer would run a sweep of virtual tests across the operating range of the pump and determine when the performance exhibits a head loss (of typically 3%) due to cavitation, at which point the NPSHR is extracted.
In summary, CFD simulations, such as those performed on the SimScale platform, can be used to assess both the NPSH available in the given system and the NPSH required for a detailed pump design. This technology enables a more accurate virtual determination of NPSHA and NPSHR and helps ensure that the margin between these two values is sufficient to avoid damaging cavitation over the life of the system.
While Net Positive Suction Head may seem like a complex engineering concept, it is actually quite simple to understand once broken down. Engineers and designers need to take NPSH into account when designing hydraulic systems and selecting the right pump for their application. CFD simulation technology enables engineers to realistically model both NPSHA and NPSHR, providing valuable insight into the pump design and selection process. With this knowledge, you can be sure that your pump will operate safely and reliably for years to come.
Last updated: August 9th, 2024
We appreciate and value your feedback.
What's Next
What Is FEA | Finite Element Analysis?Sign up for SimScale
and start simulating now