Documentation
Creep is the inelastic, irreversible deformation of solid materials during time. It is a life limiting factor for a structure and depends on variables such as stress, strain, temperature and time. This dependency can be modeled as followed:
$$ \begin{equation}\label{creep_dependency_eq}
\dot{\mathbf{\epsilon}}_{cr} = f(\sigma,\epsilon,T,t) \end{equation} \tag1$$
Creep can occur in all crystalline materials, such as metal or glass. It has various impacts on the behavior of the material, and can ultimately lead to the following problems: [NAFEMS_HT21]
Because of these extremely different factors, the effects of creep in certain situations can be highly complex. By undertaking creep analysis the influence of these effects can be evaluated and the lifetime of parts can be estimated. Especially, in the hot section of turbines of commercial and military plants, this can be a crucial factor for the design process and optimization costs.
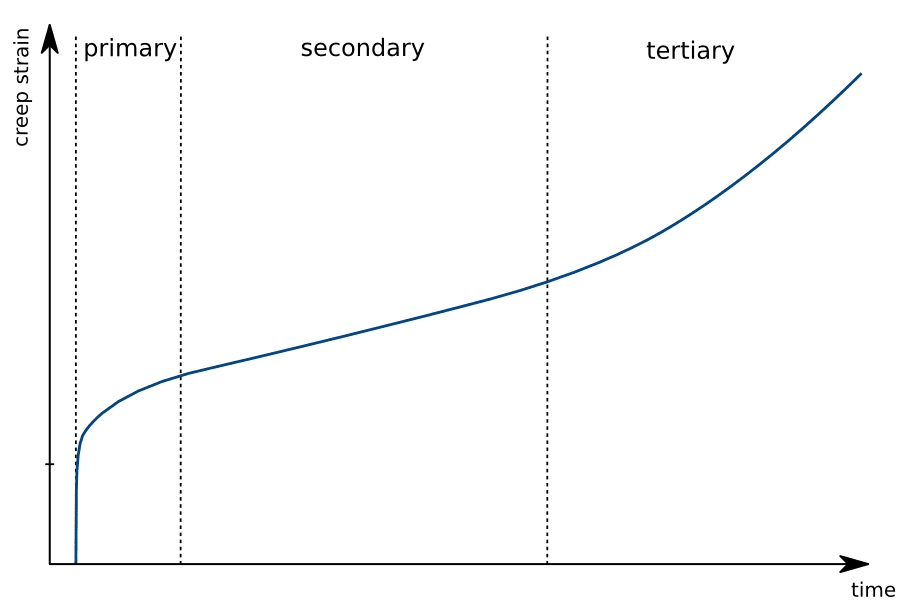
Creep can be divided into three different stages: primary creep, secondary creep and tertiary creep:
Primary creep (\(-1 < m < 0\)) starts rapidly with an infinite creep rate at the initialization. Here, \(m\) is the time index (for the strain rate form of the equations). It occurs after a certain amount of time and slows down constantly. It lasts for the first hour after applying the load and is essential in calculating the relaxation over time.
Secondary creep (\(m = 0\)) follows right after the primary creep stage. The strain rate is now constant over a long period of time.
The strain rate in the tertiary creep stage is growing rapidly until failure. This happens in a short period of time and is not of great interest. Therefore, only primary and secondary creep are modeled on the SimScale platform.
The creep strain equations are based on an additive strain decomposition:
$$ \begin{equation}\label{strain_decomposition}
\mathbf{\epsilon}_{tot} = \mathbf{\epsilon}_{el} + \mathbf{\epsilon}_{pl} + \mathbf{\epsilon}_{cr} \end{equation} $$
$$ \begin{equation}\label{small_strain_hypo} \mathbf{\sigma} = \mathbf{D} : \mathbf{\epsilon}_{el} \end{equation} $$
$$ \begin{equation}\label{creep_strain_eq} \dot{\mathbf{\epsilon}}_{cr} = \frac{3}{2} \dot{\epsilon^c} \frac {\tilde{\mathbf{\sigma}}}{\sigma_{eq}} \end{equation} $$
Where:
The elastic strain \(\mathbf{\epsilon}_{el}\) occurs right after applying the load and is connected with the stresses through the elasticity tensor \(\mathbf{D}\) in equation 1. The plastic strain \(\mathbf{\epsilon}_{pl}\) results from excessive loading and is irreversible. The creep strain tensor can be calculated with the equivalent creep strain \(\mathbf{\epsilon^c}\), the deviatoric stress \(\tilde{\mathbf{\sigma}}\) and the equivalent stress \(\sigma_{eq}\). The deviatoric stress \(\tilde{\mathbf{\sigma}}\) causes distortion of the body and results after splitting the stress tensor in additive isotropic and deviatoric components.
The fundamental creep law type defined as creep strain rate equation is available in SimScale:
Power or Bailey-Norton Law:
$$ \dot{\epsilon^c} = A \cdot \sigma^{n} \cdot t^m \cdot \epsilon^{k}$$
where we have
The creep stress index depends on the temperature and the stress level and can be determined with the help of an Ashby deformation mechanism map.
On the basis of these basic laws, three different creep formulations are currently available on the platform:
In this formulation, the creep strain rate only depends on the stresses. Therefore \(m\) and \(k\) are equal to zero:
$$ \dot{\epsilon^c} = A \cdot \sigma^{n}$$
In this formulation, the creep strain rate depends also on time:
$$ \dot{\epsilon^c} = A \cdot \sigma^{n} \cdot t^m$$
In this formulation, the creep strain rate depends on the stresses and the creep strains. (For convenience \(k\) is referred to as \(m\) on the platform.)
$$ \dot{\epsilon^c} = A \cdot \sigma^{n} \cdot (\epsilon^c)^k$$
Creep behavior can be applied to Linear Elastic and Elasto-Plastic material models. To define the model, follow the steps given below:
Supported analysis types
A creep material behavior can only be defined for the following analysis types:
It is important to have a consistent unit system when converting parameters found in literature or material supplier or test data. Often the creep parameters are given related to length measure in \(mm\), stresses in \(MPa\), and time in hours. An example of how to convert the parameter \(A\) of the Time Hardening or Strain Hardening formulation would be: \(A_{SI} = \frac{1}{3600} \cdot \frac{1}{3600^m} \cdot \frac{1}{10^{6n}} \cdot A_{MPa\;h}\)
References
Last updated: March 7th, 2024
We appreciate and value your feedback.
Sign up for SimScale
and start simulating now